Rotation des états de spin

L'espace ${\mathcal{H}}_{s}$ des états de spin, d'une particule de spin $s$ est un espace à $2s+1$ dimensions. Dans cet espace tout opérateur, et notamment tout opérateur de rotation induite, est représenté par une matrice à $2s+1$ lignes et $2s+1$ colonnes. D'une façon générale, un tel opérateur correspondant à une rotation d'un angle $\theta$ autour d'un axe $O\vec{u}$ a pour expression :

$$\mathcal{R}_u^{(s)}(\theta)=e^{-\frac{i}{\hbar}\,\theta\,S_u}= \m... ...,\theta\,S_u+ \frac{1}{2!}\,\left(-\frac{i}{\hbar}\,\theta\,S_u\right)^2+\ldots$$

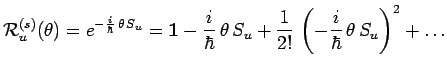Si :

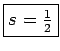Dans ce cas on pose $\vec{S}=\frac{\hbar}{2}\,\vec{\sigma}$ :

$$\mathcal{R}_u^{\left(\frac{1}{2}\right)}(\theta)= e^{-\frac{i}{\hbar}\,\theta\,S_u}= e^{-i\,\frac{\theta}{2}\,\sigma_u}$$

$$\mathcal{R}_u^{\left(\frac{1}{2}\right)}(\theta)= \mathbf{1}-i\,\... ..._u^3+\ldots+ \frac{1}{n!}\,\left(-i\frac{\theta}{2}\right)^n\,\sigma_u^n+\ldots$$

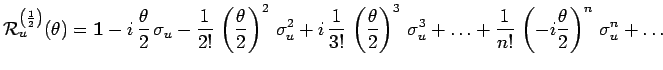Tenu compte des identités de Pauli :

$$\sigma_u^2=(\sigma_u)^{2p}=\mathbf{1}~~~~~~~~~~~~~~~~~~~~~~~~\mathrm{d'o\grave{u}}~~~~~~~~~~ \sigma_u^3=(\sigma_u)^{2p+1}=\sigma_u$$

$$e^{-i\,\frac{\theta}{2}\,\sigma_u}= \left[\mathbf{1}-\frac{1}{2}\... ...t[\frac{\theta}{2}- \frac{1}{3!}\,\left(\frac{\theta}{2}\right)^3+\ldots\right]$$

et finalement :

$$\begin{array}{\vert c\vert}\hline { }\\ ~~\mathcal{R}_u^{\lef... ...\scalebox{1.4}{$\frac{\theta}{2}$}~~\\ { }\\ \hline \end{array}$$

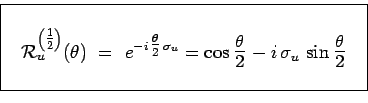et par exemple, en remplaçant les matrices $\sigma_u$ par leurs expressions explicites :

$$\mathcal{R}_x^{\left(\frac{1}{2}\right)}(\theta)= \left(\begin{ar... ...2} \\ & \\ -\sin\frac{\theta}{2} & \cos\frac{\theta}{2} \\ \end{array}\right)$$

$$\mathcal{R}_z^{\left(\frac{1}{2}\right)}(\theta)= \left(\begin{ar... ...rac{\theta}{2}} & 0 \\ & \\ 0 & e^{i\,\frac{\theta}{2}} \\ \end{array}\right)$$

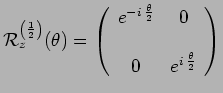Les rotations des états de spin s'obtiennent par application de ces opérateurs de rotation induite. On remarquera en particulier, que dans une rotation complète d'angle $2\pi$ , le ket ou la fonction d'onde d'une particule de spin $\frac{1}{2}$ change de signe :

$$\mathrm{Si}~~s=\frac{1}{2}~~~~~~~~~~~~~~~~~~~~~~~~\mathcal{R}_z^{2\pi}=-1$$

Si :

Les expressions des opérateurs $S_x,~S_y,~S_z$ pour une particule de spin 1 ont été données précédemment. sur ces expressions on vérifie par exemple^III14 :

$$\left(\frac{S_z}{\hbar}\right)^3=\frac{S_z}{\hbar}~~~~~~~~~~~~~~~... ...thrm{d'o\grave{u}}~~~~~~~~~~ \left(\frac{S_u}{\hbar}\right)^3=\frac{S_u}{\hbar}$$

car tout axe $O\vec{u}$ de rotation peut être choisi pour axe $Oz$ . Il en résulte :

$$\left(\frac{S_u}{\hbar}\right)^{2n}=\left(\frac{S_u}{\hbar}\right... ...~~~~~~~~~~~~~~~~~~~~~~~ \left(\frac{S_u}{\hbar}\right)^{2n+1}=\frac{S_u}{\hbar}$$

L'opérateur de rotation induite peut alors être développé suivant les puissances de l'angle $\theta$ de rotation autour de l'axe $O\vec{u}$ :

$$\begin{array}{ccl} \mathcal{R}_u^{(1)}(\theta) & = & e^{-i\,\... ...4}{4!}\,\left(\frac{S_u}{\hbar}\right)^4+\ldots \\ \end{array}$$

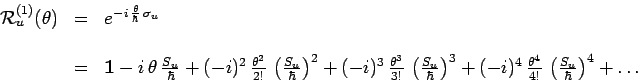ou encore, en regroupant les termes :

$$\begin{array}{ccl} \mathcal{R}_u^{(1)}(\theta) & = & \mathbf{... ...ldots\right]\, \left(\frac{S_u}{\hbar}\right)^2 \\ \end{array}$$

On reconnait les développements de $\sin\theta$ et $\cos\theta$ d'où :

$$\begin{array}{\vert c\vert}\hline { }\\ ~~\mathcal{R}_u^{(1)}... ...1.4}{$\frac{S_u}{\hbar}$}\right)^2~~\\ { }\\ \hline \end{array}$$

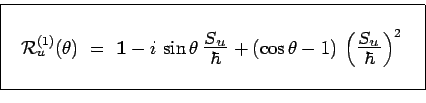et par exemple, sur la base $\{\mid +>_z,\mid 0>_z,\mid ->_z\}$ :

$$\mathcal{R}_x^{(1)}(\theta)= \left(\begin{array}{ccc} \cos^2\frac... ...-\frac{i}{\sqrt{2}}\,\sin\theta & \cos^2\frac{\theta}{2} \\ \end{array}\right)$$

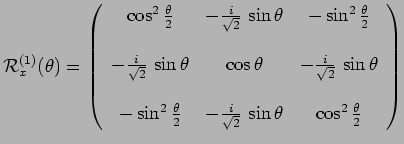Question 3-24 : Calculez les matrices :

$$\mathcal{R}_y^{(1)}(\varphi)~~~~~~~~~~~~~~~~~~~~~~~~\mathrm{et}~~~~~~~~~~~~~~~~~~~~~~~~ \mathcal{R}_z^{(1)}(\psi)$$

Question 3-25 : Une rotation quelconque dans l'espace peut être réalisée en effectuant successivement les trois rotations suivantes :

$\imath-$ rotation d'angle $\alpha$ autour de $O\vec{z}$ ($O\vec{y}$ vient en $O\vec{u}$ ),

$\imath\imath-$ rotation d'angle $\beta$ autour de $O\vec{u}$ ($O\vec{z}$ vient en $O\vec{Z}$ ),

$\imath\imath\imath-$ rotation d'angle $\gamma$ autour de $O\vec{Z}$ ($O\vec{u}$ vient en $O\vec{Y}$ ),

soit :

$$\mathcal{R}(\alpha\beta\gamma)=\mathcal{R}_Z(\gamma)\,.\,\mathcal{R}_u(\beta)\,. \,\mathcal{R}_z(\alpha)$$

où $\alpha,~\beta,~\gamma$ sont les angles d'Euler de la rotation $\mathcal{R}$ . Démontrez :

$$\mathcal{R}(\alpha\beta\gamma)=e^{-i\,\alpha\,J_z}\,.\,e^{-i\,\beta\,J_y}\,.\, e^{-i\,\gamma\,J_z}$$